Warning: Undefined variable $ext in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/show-hidecollapse-expand/bg_show_hide.php on line 281
Warning: Undefined variable $ext in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/show-hidecollapse-expand/bg_show_hide.php on line 281
Warning: Undefined variable $ext in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/show-hidecollapse-expand/bg_show_hide.php on line 281
7. 93 m is equal to _______ km.
A. 0.093
B. 9.3
C. 93,000
D. 9,300
Below is a chart of the metric prefixes most commonly used from smallest (milli) to largest (kilo).
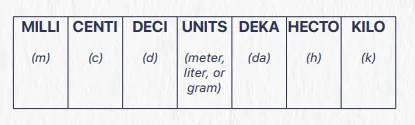
The Rules:
For every step you move to the right, you divide your number by that power of ten.
For every step you move to the left, you multiply your number by that power of ten.
8. Solve 3\frac{5}{8} × 2\frac{2}{5}
A. 6\frac{1}{4}
B. \frac{10}{87}
C. 8\frac{7}{10}
D. 7\frac{9}{16}
9. On a recent road trip, Orlando drove an unknown number of miles during his first week. He then drove fifteen more miles in his second week and twenty-five fewer miles in his third week when compared to the first week. Write an expression that represents the total number of miles that Orlando drove in the
3-week road trip.
A. 3x – 40
B. 3x + 10
C. 3x + 40
D. 3x – 10
Warning: Undefined variable $in_same_cat in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/EXP.GKFEED.COM/function.php on line 27
Warning: Undefined variable $excluded_categories in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/EXP.GKFEED.COM/function.php on line 27
