6. A particular company produces 500 computers a day. For 20 days the number of defective computers produced each day was recorded, and the results were placed in the table below.

Based on this data, on average over a long period of time, what is the expected number of defective computers produced on any given day?
A. 0.45
B. 0.75
C. 1
D. 1.5
E. 2.25
7. The function f(x) when graphed in the standard (x,y) coordinate plane has the features below:
I. One of its intercepts is located at (−3,0).
II. f(x) increases for all x > 3.
III. f(x) is not defined for x = −2.
One of the following is the graph of f(x). Which one?
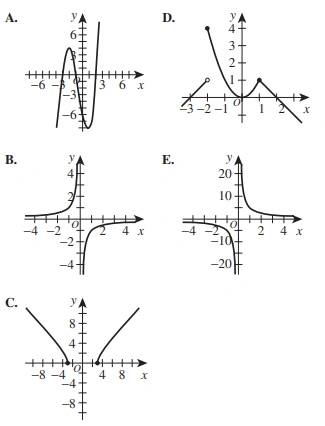
8. In ΔPQR shown below, r = 8 meters, p = 10 meters, and the measure of ∠Q is 120°. The solution of which of the following equations gives the length q in meters?
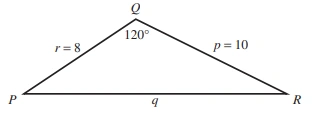
(Note: For a triangle with sides of length a, b, and c that are opposite angles ∠A, ∠B, and ∠C, respectively, \( \frac{sin∠A}{a}=\frac{sin∠B}{b}=\frac{sin∠C}{c} \) and c² = a² + b² − 2ab cos∠C.)

9. Consider the 4 expressions below, where m and n are distinct integers greater than 2.
\[ \frac{m}{n-1}, \frac{m}{n},\frac{m}{n+1},\frac{m-1}{n} \]
If it can be determined, which of the 4 expressions must have the greatest value?

10. The original price of an item was decreased by 20%. The 1st reduced price was decreased by 20% and then that 2nd reduced price was decreased by 50%. The price that resulted from these 3 decreases was what percent less than the original price?
F. 10%
G. 32%
H. 68%
J. 90%
K. 98%