Warning: Undefined variable $ext in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/show-hidecollapse-expand/bg_show_hide.php on line 281
Warning: Undefined variable $ext in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/show-hidecollapse-expand/bg_show_hide.php on line 281
Warning: Undefined variable $ext in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/show-hidecollapse-expand/bg_show_hide.php on line 281
Warning: Undefined variable $ext in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/show-hidecollapse-expand/bg_show_hide.php on line 281
Warning: Undefined variable $ext in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/show-hidecollapse-expand/bg_show_hide.php on line 281
The 2024 SAT standardized test is a critical assessment for college admissions. The math section covers a wide range of topics. To help students prepare, we’ve created a practice paper. This paper includes various math problems to help students become familiar with the test format and excel in their exam.
1.
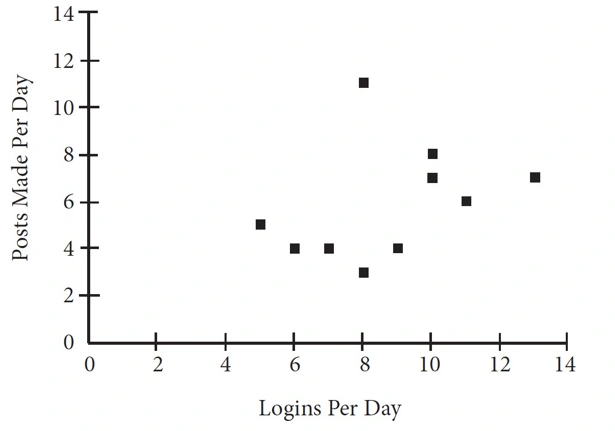
Given the scatterplot graph above, ten students at Welton Academy were polled at random about their usage of the school’s new physics-centered social media app, E = MC Shared. The app was developed to encourage students to discuss physics curricula and concepts in ways that mirrored social media trends in 2013. Students were asked how many times they logged into the app each day as well as how many posts they actually made using the app. With the given data, what conclusions can be drawn about this group of students?
A. The majority of students polled logged in more times per day than they posted.
B. The majority of students polled posted more times per day than they logged in.
C. The majority of students polled logged in and posted an equal number of times.
D. No relationship can be drawn between logins per day and posts per day.
2.

Two graphs, f(x) and h(x), are shown above. If f(x) = 3x + 4 and f(x) and h(x) are perpendicular, which of the following could be the equation of h(x) ?
A. h(x) = \frac{1}{3} x + 9
B. h(x) = -\frac{1}{3} x + 9
C. h(x) = 3x + 9
D. h(x) = -3x + 9
3. The number of eggs that Farmer Jones has in his chicken coop will grow exponentially as Farmer Jones buys more chickens to increase production. The number of eggs Farmer Jones has in the coop can be modeled by the equation y = 3^x beginning on Day 1, where x is given by x = 1, and y is the number of eggs currently in the coop. If the coop can support only 4,000 eggs, and Farmer Jones empties the coop every day, on which day will the chickens produce too many eggs for the coop to support?
A. Day 6
B. Day 7
C. Day 8
D. Day 9
4. If a = \frac{4a²}{16} and a is a nonzero integer, which of the following is equivalent to a ?
A. 4a
B. 4 \sqrt[]{a}
C. \sqrt[]{2a}
D. 2 \sqrt[]{a}
5. Three different chefs work together to prepare meals for 280 dinner guests. Each works at a different speed, and their combined output throughout the night is modeled by the equation 8x + 4x + 2x = 280. If x is a positive integer, which of the following could 8x represent in the equation?
A. The total meal output by the slowest chef, who made 40 meals.
B. The total meal output by the fastest chef, who made 160 meals.
C. The total meal output by the fastest chef, who made 80 meals.
D. The difference between the output between the slowest and fastest chef, which would be 120 meals.
Warning: Undefined variable $in_same_cat in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/EXP.GKFEED.COM/function.php on line 27
Warning: Undefined variable $excluded_categories in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/EXP.GKFEED.COM/function.php on line 27