6. Taylor is 6 feet tall. If 1 foot is equal to approximately 0.3 meters, then which of the following is closest to Taylor’s height in meters?
A. 1.8
B. 2
C. 18
D. 20
7.
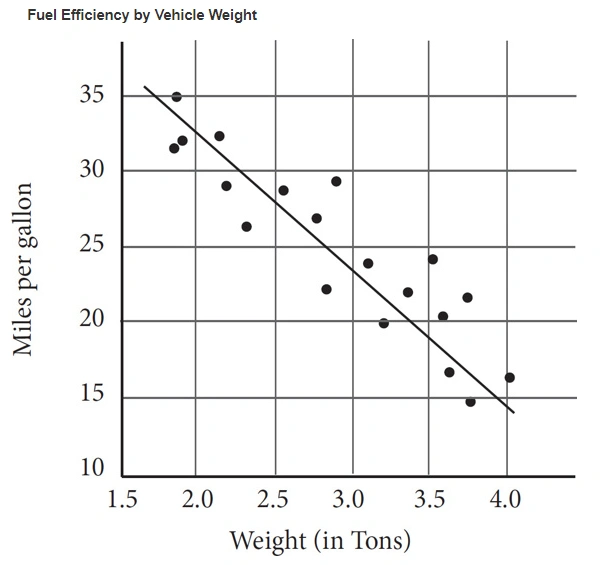
The scatterplot above shows the fuel efficiency, in miles per gallon, of a variety of vehicles weighing between 1.5 and 4 tons. Based on the line of best fit to the data represented, which of the following is the closest to the expected miles per gallon of a vehicle that weighs 3 tons?
A. 20
B. 24
C. 27
D. 28
8.
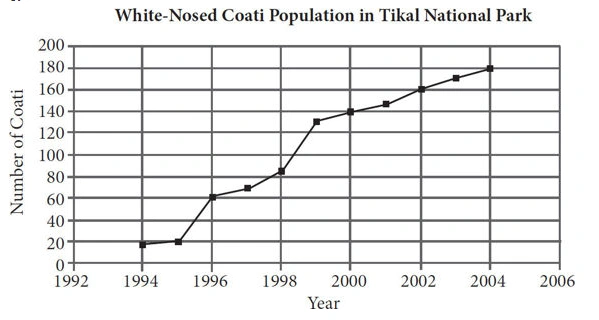
As part of a recent wildlife conservation effort in Guatemala, park rangers in Tikal National Park have tracked the growing number of white-nosed coati living within a certain protected region over the period 1994-2004.
According to the data above, if the population of coati in the protected region of Tikal National Park increased at the same rate from 2004-2006 as it did from 2000-2004, then what was the number of coati in the park in 2006 ?
A. 180
B. 190
C. 200
D. 210
9.
\[ \frac{8d+10-d}{3}=\frac{d(3+4)+a}{3} \]
If the equation above has infinitely many solutions for d, what is the value of a ?
A. -10
B. 10
C. 15
D. 20
10.
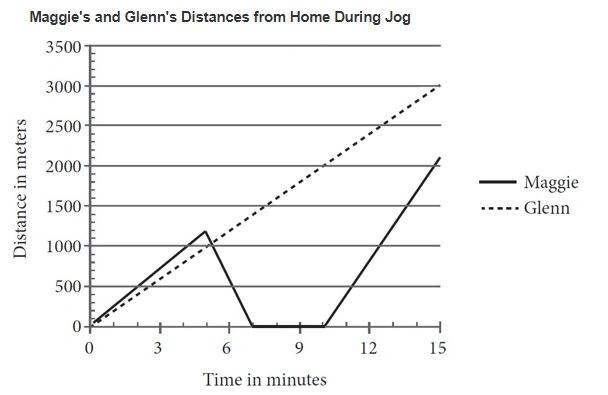
Maggie and Glenn both leave from the same house to go for a jog along a trail. Shortly after leaving, Maggie realizes she forgot her iPod and returns home to find it before heading back out onto the same trail. The graph above shows how far each of them is from home for the first fifteen minutes of their jogs. What is Glenn’s approximate average speed in meters per second for the portion of his jog shown?
A. 3.3
B. 15
C. 200
D. 12000