2024 SAT Test Math Practice 18


Warning: Undefined variable $ext in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/show-hidecollapse-expand/bg_show_hide.php on line 281

Warning: Undefined variable $ext in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/show-hidecollapse-expand/bg_show_hide.php on line 281

Warning: Undefined variable $ext in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/show-hidecollapse-expand/bg_show_hide.php on line 281

Warning: Undefined variable $ext in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/show-hidecollapse-expand/bg_show_hide.php on line 281

Warning: Undefined variable $ext in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/show-hidecollapse-expand/bg_show_hide.php on line 281

The 2024 SAT standardized test is a critical assessment for college admissions. The math section covers a wide range of topics. To help students prepare, we’ve created a practice paper. This paper includes various math problems to help students become familiar with the test format and excel in their exam.

 

1. In a certain sporting goods manufacturing company, a quality control expert tests a randomly selected group of 1,000 tennis balls in order to determine how many contain defects. If this quality control expert discovered that 13 of the randomly selected tennis balls were defective, which of the following inferences would be most supported?

A. 98.7% of the company’s tennis balls are defective
B. 98.7% of the company’s tennis balls are not defective
C. 9.87% of the company’s tennis balls are defective
D. 9.87% of the company’s tennis balls are not defective

Correct Answer: B

Answer Explanation:

The quality control expert discovered that 13 out of 1,000 randomly selected tennis balls were defective. ​\( \frac{13}{1000} \)​= 0.013, which is equivalent to 1.3%. This means that 100 – 1.3 = 98.7% of tennis balls tested were not defective, and this data most supports answer (B).

Advertisement

2. If ​\( -\frac{20}{7} \)​ < -3z + 6 < ​\( -\frac{11}{5} \)​ , what is the greatest possible integer value of 9z – 18 ?

A. 6
B. 7
C. 8
D. 9

Correct Answer: C

Answer Explanation:

Sat math 28 1

Advertisement

3. -24 – 8j = 12k
3 + ​\( \frac{5}{3}k \)​ = ​\( -\frac{7}{6}j \)​

Which of the following ordered pairs (j, k) is the solution to the system of equations above?

A. (6, -6)
B. (3, 0)
C. (0, 2)
D. (-4, 1)

Correct Answer: A

Answer Explanation:

Whenever there are variables in the question and numbers in the answer choices, think Plugging In the Answers. In (A), j = 6, and k = -6. Plug these two values into the first equation to get -24 – 8(6) = 12(-6). Solve for both sides of the equation to get -24 – 48 = -72, or -72 = -72. Therefore, the values work for the first equation. Plug the values into the second equation to get 3 + \( \frac{5}{3} \)(-6) = \( -\frac{7}{6} \)(6). Solve both sides of the equation to get 3 + (-10) = -7, or -7 = -7. Since the values given in (A) work in both equations, the correct answer is (A).

Advertisement

4. United States Investment in Alternative Energy Sources

Sat math 28 2

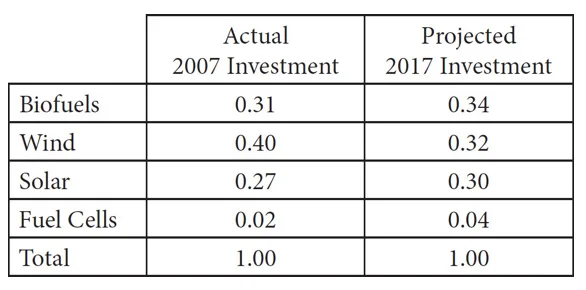
The table above shows the relative investment in alternative energy sources in the United States by type. One column shows the relative investment in 2007 of $75 million total invested in alternative energy. The other column shows the projected relative investment in 2017 given current trends. The total projected investment in alternative energy in 2017 is $254 million. Suppose that a new source of alternative energy, Cold Fusion, is perfected. It is projected that by 2017 that $57 million will be invested in Cold Fusion in the United States, without any corresponding reduction in investment for any other form of alternative energy. What portion of the total investment of alternative energy in the United States will be spent on biofuels?

A. 0.18
B. 0.22
C. 0.28
D. 0.34

Correct Answer: C

Answer Explanation:

First, you know the new proportion must be less than the current 0.34 for biofuels (because the total amount spent on alternative energy is increasing, but the amount spent on biofuels is remaining the same), so you can eliminate (D). Next, determine the amount that will be spent on biofuels in 2017 by multiplying 0.34 by the total of $254 million: 0.34 × 254 = $86.36 million. Because 57 million new dollars will be spent on alternative energy, the new total will be 254 + 57 = $311 million. Divide $86.36 million by $311 million to get the new proportion: ​\( \frac{86.38}{311} \)​= 0.28, which is (C).

Advertisement

5. (x – 2)² + y² = 36
y = -x + 2

The equations above represent a circle and a line that intersects the circle across its diameter. What is the point of intersection of the two equations that lies in quadrant II?

Sat math 28 3

Correct Answer: D

Answer Explanation:

In quadrant II, the x-coordinate is negative, and the y-coordinate is positive. Therefore, eliminate (C). Whenever the question includes variables and the answers are numbers, think Plugging In the Answers. Of the remaining answers, (B) is easiest to work with. In (B), the x-value is -4 and the y-value is 2. Plug these values into the second equation to get -4 = -2 + 2. Given that this is not a true statement, eliminate (B). Try the values in (A) in the second equation to get 3\( 3\sqrt[]{2} \)​ = -(​\( -3\sqrt[]{2} \)​) + 2. This is also not true, so the correct answer is (D).

Advertisement
Pages: 1 2

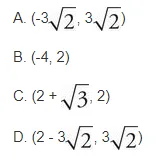
Warning: Undefined variable $in_same_cat in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/EXP.GKFEED.COM/function.php on line 27

Warning: Undefined variable $excluded_categories in /www/wwwroot/gkfeed.com/public_html/wp-content/plugins/EXP.GKFEED.COM/function.php on line 27
< PREVIOUS POST2024 SAT Test Math Practice 17
NEXT POST >2024 SAT Test Math Practice 19

Leave A Comment?